NON - LINEAR STRUCTURAL ANALYSIS

Non - Linear Structural Analysis

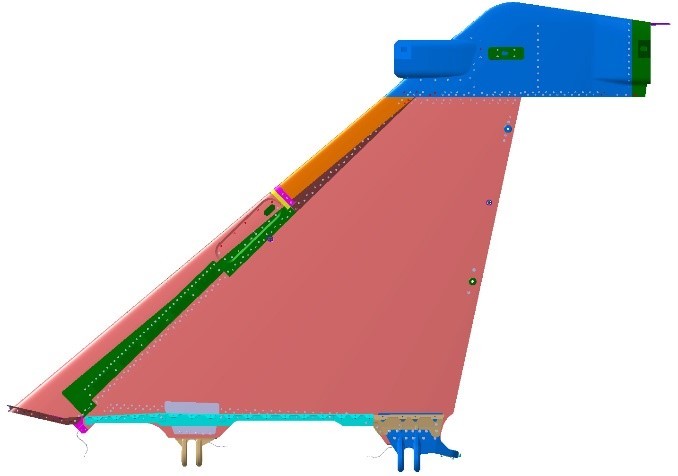
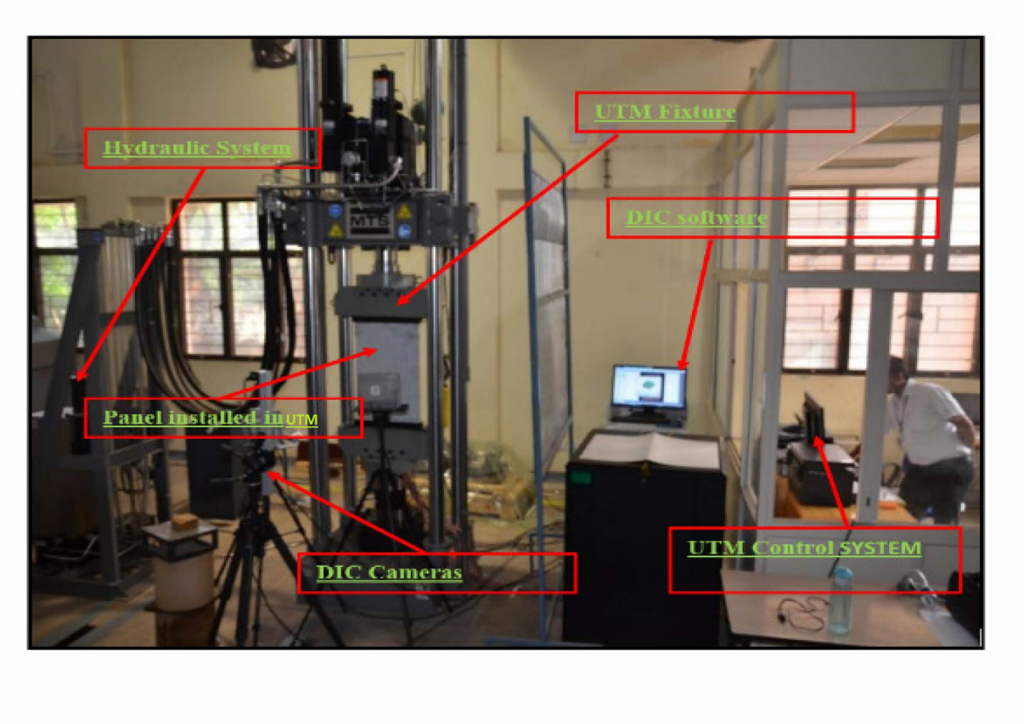
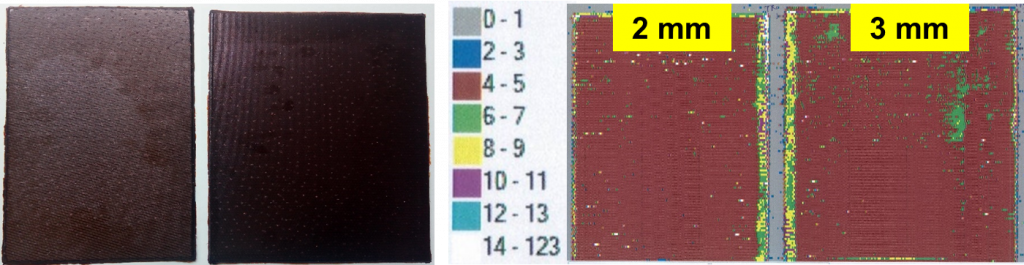
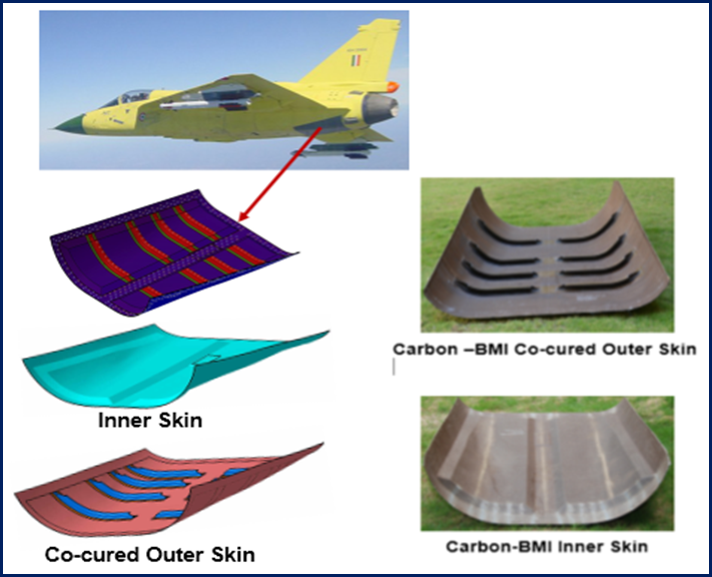
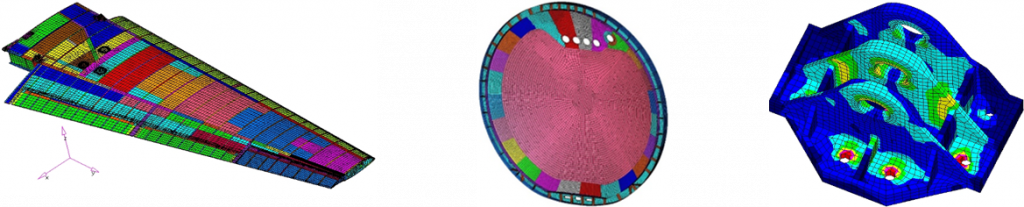
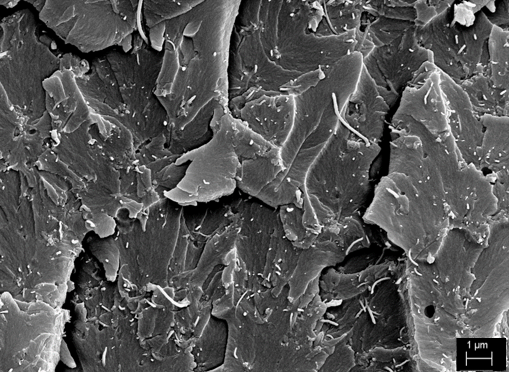
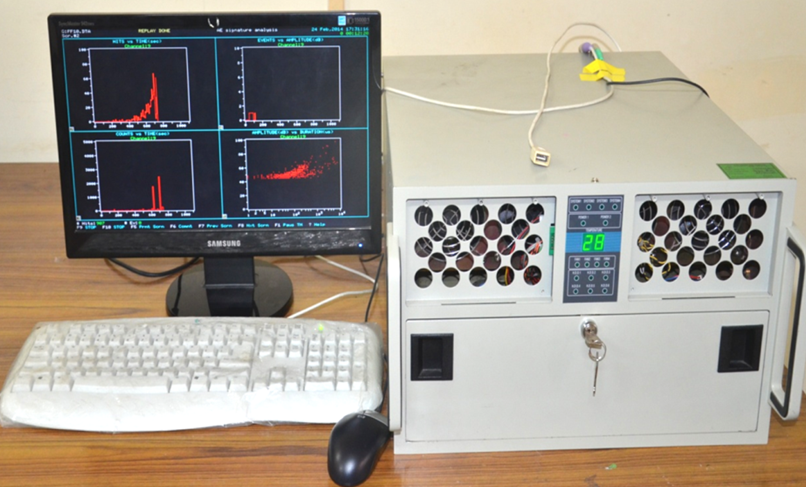
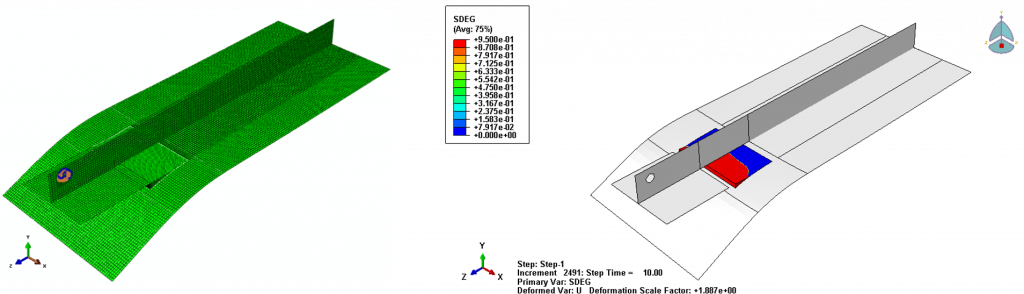
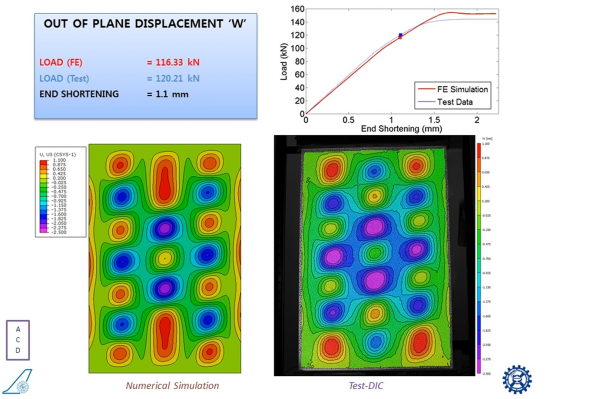
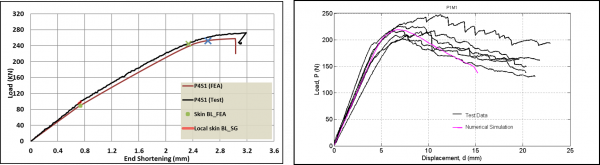
ACD is immensely involved in finite element solution techniques for providing solutions for wide range of static, dynamic, buckling, optimization and non-linear (both geometric and material) analysis problems encountered in aerospace composite design and analysis. Accurate and efficient analysis of composite structures using state-of-the-art solution methods, convergence techniques and time integration schemes have been used to provide best possible design to the aerospace industries. Efficient modeling and non-linear solution techniques are developed to solve post-buckling and damage tolerance problem where various conditions such as large displacement, onset of damage/crack, non-linear behaviour, damage modes, damage location and the collapse of the structure are captured accurately. With the advent of the digital computer and finite element tools which not only speed up calculations but also allow display of results in graphics, commercial finite element tools such as Abaqus, HyperMesh, MSC Nastran has been utilized for solving complex problems. Modified Newton-Raphson method and Arc-length methods, which facilitate the tracing of the load-configuration path beyond buckling points, are systematically examined to provide solutions for non-linear problems. Specific emphasis is focussed on obtaining the complete structural response in an efficient and reliable way. The inter-laminar and intra-laminar progressive damage in composites due to static or dynamic loads are captured by using appropriate damage models.

The nonlinear analysis methodologies have certainly developed due to the need for the use of optimized structures and the use of new designs that are being introduced or have been introduced over the recent years in the division. Moreover, for addressing of damage tolerance design of structures there is more rigorous attention being given to such damage tolerance related issues wherein the developed non-linear solutions are helping to move closer to the best possible designs.